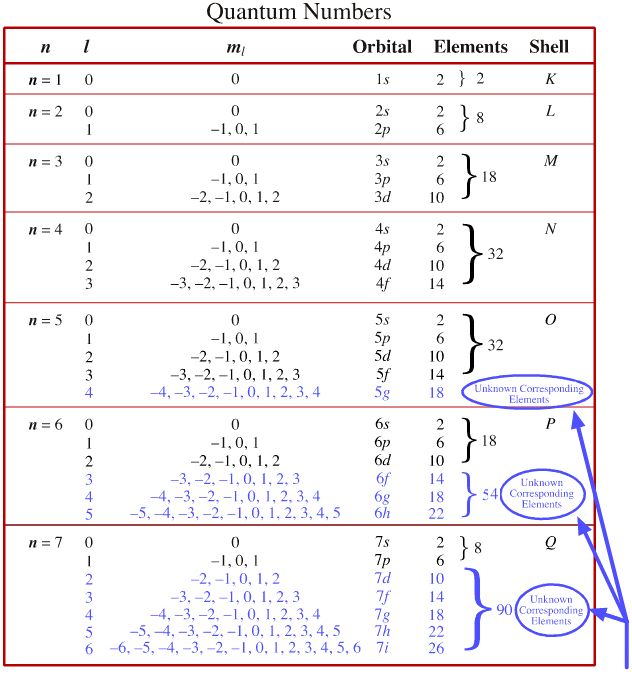
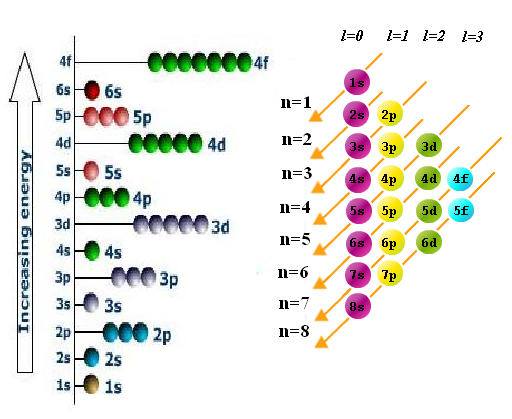
Graphing Atom Orbital Calculator Program
Yes, there are equations, they are called spherical harmonics. They are given here (look at equations 18 through 33): In my opinion, if you are not interested in the math of QM it isn't worth dealing with them, it would be better to print out a piece of paper with the pictures on it. QM math does look rather difficult to solve and Understand it too for me, but I'm so interested in calculating the probability of finding an electron of a given distance from the nucleus I just thought that maybe for somebody inexperienced as I am would just be given somekind of formula together with a introductory tutorial explaining the physics behind the formula---made easy for me to understand withought all the technical terms. Word 2010 Folded Booklet Template. I am just interested in the qualitative understanding behind orbital shapes and calculating the probability of finding electrons at a given distance from the nucleus (if there is a formula(s) for that). That's pretty much the idea behind my post.
I want an understanding in simple bunny-easy terms. LOL i know i sound like an idiot but i just completed Grade 12 and with no knowledge or familiarity of what is harmonics or whatever I deserve an explanation in very simple terms. I remember my chem teacher in class saying, 'the electron dematerializes into radiation and then rematerializes back into matter just like star treck' I was so overwhelmed by that i spent endless days trying to picture how electrons show complimentarity of waves and particles. That's the whole reason why i wanted to learn orbitals and all that neat stuff. If there are any resources available like courses or online tutorials or pretty much ANYTHING out there that can help me understand princples and dynamics of orbitals I'd be filled with delight. One question: Whenever i think about wave-particle duality is it reasonable to picture it like this? A wave packet that has energy concentrated in one fraction of space-time but thats only if picturing a hypothetical 'eye' close-up view.
Molecular orbitals editor. By selecting Orbital/Peak Selection tool and clicking on the molecular orbital energy level of interest you will see in AO.
Now, take the eye away from the 'wave-packet' has to increase the dimension of space-time and the 'wave-packet' pictures so concentrated in space-time that to us now, in the new 'zoomed-out' view of the dimension the wave packet seems like a localized particle but is actually a small little wave concentrate and tightly packed with energy----some sort of energy i do not know what to call it appropriately but some form of energy indeed. Am i correctly picturing wave-particle duality in my sense?
Or is there another view which is more correct but translates into the same picture as mine? Is the virial theorem true for atoms and molecules? It says: The total energy (kinetic + potential) of an electron in an atom or a molecule is always one-half its potential energy.
Thus, for example, when an electron is shifted from a 1s to a 2s orbital, its potential energy increases by 3.27 aJ. At the same time the electron slows down and its kinetic energy drops by half this quantity, namely, 1.635 aJ.
The net result is that the total energy (kinetic + potential) increases by exactly half the increase in potential energy alone; i.e., it increases by 1.635 aJ. A similar statement can he made for any change inflicted on any electron in any atomic or molecular system. This result is known as the virial theorem. Because of this theorem we can, if we want, ignore the kinetic energy of an electron and concentrate exclusively on its potential energy. Now that is just wierd. I always thought that atomic orbitals are independent of each other.